M.C. Escher's four circle limit
drawings:

Circle limit 1 |

Circle limit 2 |

Circle limit 3 |

Circle limit 4 |
Here is what
M.C.Escher wrote to his son Arthur in 1960 (speaking about Circle limit 3):
" Ik heb mij rot gewerkt om
eindelijk die litho af te maken en vervolgens, de tanden op
elkaar, vier dagen lang nog eens negen mooie afdrukken van die hoogst
bewerkelijke cirkellimiet-in-kleuren gemaakt. Elke druk bestaat uit een
serie van twintig maal afdrukken: vijf planken, elke plank vier keer.
Dit alles met het werkwaardige gevoel dat dit werkstuk een mijlpaal in
mijn ontwikkeling betekent en dat er nooit iemand zal zijn, behalve
ikzelf, die dat zal inzien."
( " I worked terribly hard
to finally finish that litho, and then with gritted teeth, spent
another four days making beautiful prints of that extremely complex
circle limit in colors. Each print is a series of twenty printings:
five pieces, and each piece four times. All this with the remarkable
feeling that this work is a milestone in my development, and that
nobody, except myself, will ever realize this." )
(From: De magie van M.C.Escher. Taschen
Uitgeverij, Keulen 2003)
He was wrong when he said that nobody would realize the importance of these works, but he was certainly right in saying that the creation of such drawings by hand is extremely difficult and tiresome, much more so than plane (Euclidian) tilings. M.C. Escher made over a hundred such tilings, and something that he most probably never knew is that some of them (not all) can be transformed to hyperbolic, "circle limit", tilings with the help of a computer.
The images below were created starting from relatively low resolution
images of original Escher prints, and are therefore not perfect. In
some cases the lines where the basic tile was cut can clearly be seen.
Where this is the case, it is due in part to the quality of the
original image file, and in part to some inaccuracy both on my part and
on M.C.Escher's part.
It is an open question how M.C. Escher's work would have been
influenced had computers been available in his lifetime.
All the images below were created using Ultrafractal.

E15 by M.C.Escher |

|

E70 by M.C.Escher |
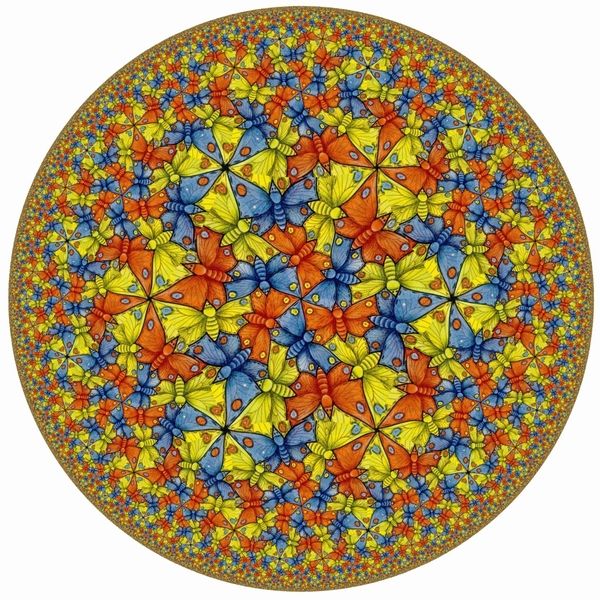
|

E66 by M.C.Escher |

|

E42 by M.C.Escher |

|

E35 by M.C.Escher |
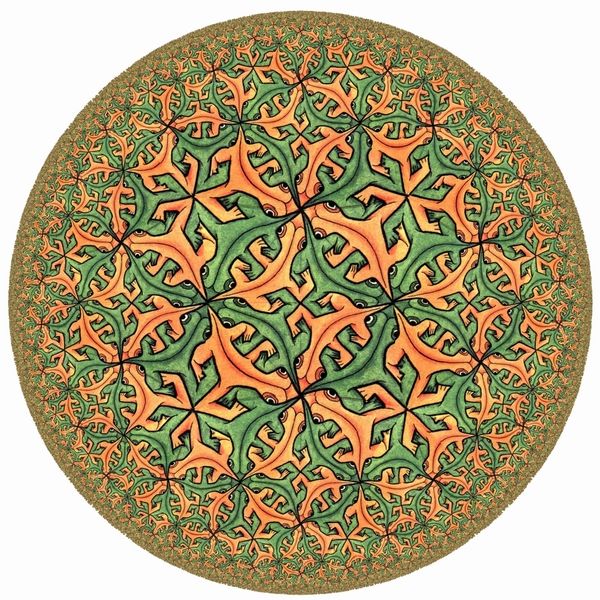
|

E57 by M.C.Escher |

|

E67 by M.C.Escher |

|

E61 by M.C.Escher |

|

E104 by M.C.Escher |

|

E105 by M.C.Escher |

|

E45 by M.C.Escher |

|

E55 by M.C.Escher |

|

E69 by M.C.Escher |
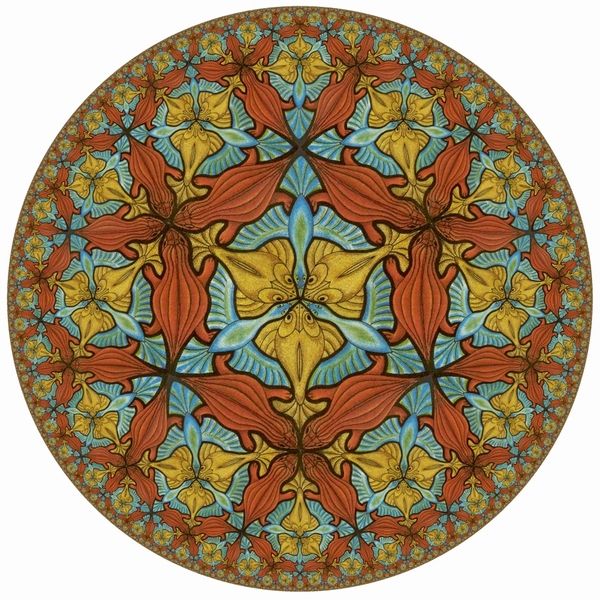
|

E85 by M.C.Escher |

|

E119 by M.C.Escher |

|

E99 by M.C.Escher |

|

E94 by M.C.Escher |
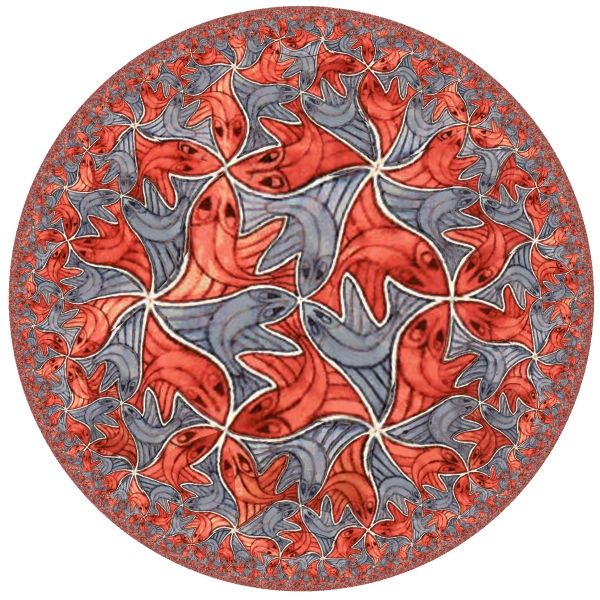
|

E103 by M.C.Escher |

|

E44 by M.C.Escher |

|

E128 by M.C.Escher |

|

E124 by M.C.Escher |

|
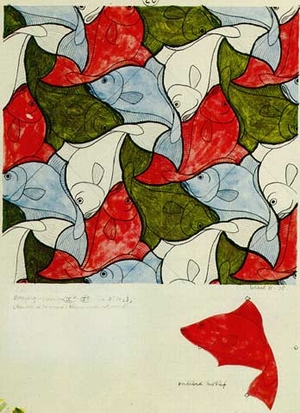
E106 by M.C.Escher |

|

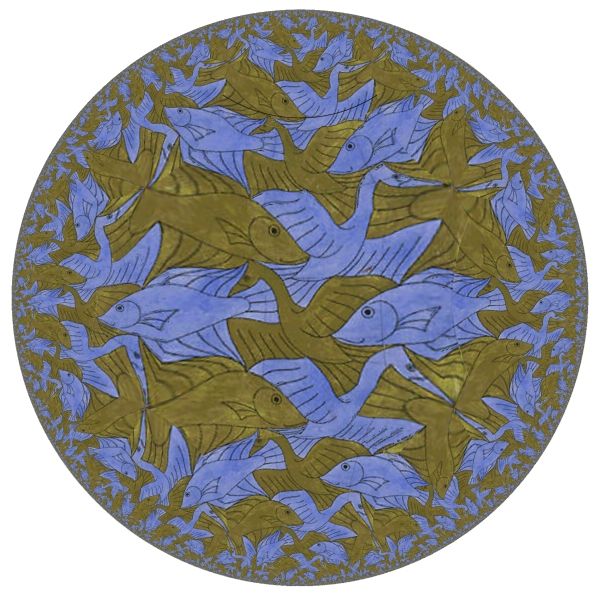
E34 by M.C.Escher |
|

E34B by M.C.Escher |

|

E58 by M.C.Escher |

|

E62 by M.C.Escher |

|

E63 by M.C.Escher |

|

E82 by M.C.Escher |

|

E123 by M.C.Escher |

|
Some transformations of other Escher
works
|

Unknown title by M.C.Escher |

|

Tetraedral planetoid by M.C.Escher |

|
| |



